| Vocademy |
Electromagnetic Waves
What are waves
In electronics, the term "wave" means two different phenomena.
Voltage waves
First, let's look at an oscillator. This is a circuit that produces a voltage that is continually changing. Let's say a particular oscillator starts at negative 10 volts and then flips to positive 10 volts 100 times every second. This is called a square wave. It is called a square wave because if we measure this voltage on an oscilloscope, we see a trace that has square corners.
 |
| The lower trace on this oscilloscope is a square wave[1]. This one is a bit rounded on the leading edge due to capacitance or inductance in the circuit being measured. |
Let's
say the oscillator produces a voltage that looks like a spiral viewed from one
side. This is called a sine wave. The upper trace in the previous illustration
is a sine wave, although it is rich in certain harmonics that make it appear
like a triangle wave with rounded apexes.
Such voltage waves are said to
have different shapes based on their appearance on an oscilloscope. However,
they are not waves in the context of what most people think of as waves. They
are not something physical like waves on the ocean or sound waves. They are
merely a changing voltage.
Energy waves
The other kind of wave is a physical thing. When most people think of waves,
they think of waves like those on the ocean or sound waves in the air. This kind
of wave is a tradeoff between potential energy and kinetic energy.
Let's
say you are on a ship on the ocean, and a series of waves are coming from a
distant storm. The ship will ride over the waves as they pass. When the ship is
at the crest of a wave, it has potential energy. At the moment, it is held up by
the wave but will soon fall as the wave passes. While it is held up, it has the
potential to fall but will not fall until the wave passes. While falling, it has
kinetic energy—the energy of motion. It will have its greatest kinetic energy as
it passes the midpoint between the crest and the next trough. When it reaches
the trough, it will have potential energy again. This time it has the potential
to go upward when the next wave lifts it. It will once again have the greatest
kinetic energy as it passes the midpoint between the trough and the next crest.
Notice that the ship only borrows the energy as the wave passes. The energy
moves on with the waves.
Making waves
Oscillators make voltage waves. These circuits create a continually changing
voltage with a particular shape, as described above.
Energy waves are
created when you disturb the equilibrium of some medium that waves can pass
through. In the case of ocean waves, let's scale it down and look at a pond on a
calm morning. When the pond's surface is glassy smooth, it is in equilibrium;
gravity pulls the water downward, and hydrostatic pressure pushes it upward. The
two forces are balanced. Now, let's throw a rock into the pond. As the rock
penetrates the surface of the water, it drags some water with it. It also pushes
water to the side in all directions. This water that is pushed away from the
rock is forced upward into a ring of water above the surface.
 |
| Drops in milk in slow motion show how energy waves form. |
As
described above, this ring has potential energy. That energy is moving away from
the point where the rock hits the water. These waves of energy move along the
water's surface in all directions. Note that the water remains stationary,
except for the up and down motion. However, the energy moves on, away from the
disturbance made by the rock. If we follow one of the crests, we will see a wave
of potential energy radiate away from the disturbance. However, the water does
not move except for the up and down motion as the wave goes by. This is called a
gravity wave (not to be confused with cosmic gravity waves, which we will not
attempt to describe here).
There are other kinds of waves. For example,
the air is in equilibrium if you are in a quiet room. However, move some of that
air, and you create a disturbance in that equilibrium; we get a higher air
pressure where air molecules are forced closer together and a lower air pressure
where they are allowed to move apart. The higher pressure acts on the air around
it, causing a pressure wave to move away from the disturbance. Here we also have
a tradeoff between potential energy and kinetic energy. Where the air has a
higher pressure (because it is forced into a smaller space), it has the
potential to expand and have less pressure. Where the pressure is lower (because
it has been allowed to expand into a larger space), it has the potential to be
compressed into a higher pressure by the surrounding high-pressure air. When
that air is in motion, either being compressed or being allowed to expand, it
has kinetic energy.
 |
| An illustration of sound waves created by an expanding and contracting sphere[2]. |
The
above illustration shows a sphere that expands and contracts rhythmically. When
it expands, it pushes air molecules closer together. When it contracts, it pulls
them apart. This creates expanding rings of compressed and rarified air that
radiate away from the sphere in all directions. Notice that any particular air
molecule moves back and forth while the sound waves pass. As the air molecules
move back and forth, they alternate between having potential and kinetic energy.
The sound waves carry this energy away from the sphere.
Let's go back to
the surface waves on the water. The rock drags water down with it. Once the rock
is gone, we are left with a conical depression in the water's surface.
Hydrostatic pressure pushes this water upward again to the same level where it
started. This gives the water at the bottom of the depression potential energy.
As the water is pushed upward, it gains kinetic energy. As the water passes the
pond surface level, it has the maximum kinetic energy and overshoots to create a
mound of water. Gravity pulls this mound downward, giving it potential energy.
The mound will fall, gaining kinetic energy, passing the surface level to create
another depression. This process will repeat until the energy imparted by the
rock is dissipated.
A bit of terminology
Before we move on, let's learn some terms to describe waves.
Frequency
WWe have a quantity that changes periodically with any wave, voltage, or energy waves. For example, let's say a voltage wave changes from +10 volts to -10 volts and back 100 times per second. The number of times it repeats this cycle over a specific period is called the frequency. Frequency is usually measured in Hertz or cycles per second (they are the same thing). So if the wave goes through the cycle 100 times per second, we call that 100 cycles per second or 100 Hz.
Wavelength
Energy waves move through a medium. Surface waves move across the surface of a pond, other bodies of water, or solid surfaces. Sound waves move through the air or other material. Let's say we are watching surface waves go by, and we notice that the crests of the waves are 10 feet apart. This distance is the wavelength; the wavelength is 10 feet. A sound wave may have the areas of highest pressure a few feet apart or a few inches apart. This would be the wavelength of the sound wave. We measure the wavelength with an electromagnetic wave as the distance between the areas where the voltage is of the same strength and polarity.
For example, let's say you freeze time and find the position where the electric field is strongest. Let's also say that a ladder is at this position, and you climb upward. On the ladder, you find that the voltage is higher. Likewise, if you go downward, the voltage decreases. Back to ground level, as you walk further into the wave, you find the electric field weaker until you reach a position where you can no longer detect an electric field. You continue walking, and the voltage gets stronger again. However, if you climb a ladder this time, the voltage becomes lower. Likewise, if you go lower, the voltage becomes higher. This gradient reversal indicates that the voltage is of the opposite polarity as before. Eventually, you reach a position where the electric field is as strong as the previous strongest position but with the polarity reversed. Walk on, and the field again gets weaker and weaker until it disappears. As you continue, the field reappears back at its original polarity. You keep walking and find yourself at a position identical to the first; the voltage is strongest and becomes higher if you climb upward and lower as you go downward. The distance between these identical positions, where the electric field is strongest and of the original polarity, is the wave's wavelength. If the distance between these positions is 10 feet, the wavelength is 10 feet.
Amplitude
The amplitude of a voltage wave is its voltage. Amplitude can be expressed in several ways. The three most common are peak, peak-to-peak, and RMS. The peak voltage is the maximum deviation from the mean. Peak-to-peak measures the greatest deviation from the highest to the lowest voltage. If the wave is centered on zero volts, this will be from the greatest positive voltage to the greatest negative voltage. RMS (root-mean-square) is a mathematical representation of a DC voltage that carries the same energy as the changing voltage. The amplitude of a surface wave is its height. This can be measured from the mean to the top of a crest (peak amplitude) and from trough to crest (peak-to-peak). RMS is not used to express the amplitude of surface waves. The amplitude of an electromagnetic wave is an expression of the strength of the electric field.Electromagnetic waves
Around 1832, James Clerk Maxwell hypothesized that
light consisted of waves of energy that consisted of electric and magnetic
fields. He also developed a mathematical analysis of these waves. The wavelength
of visible light is very short, from 400 to 700 nanometers (0.0000004 to
0.0000007 meters). His mathematical work on light predicted the existence of
such waves at longer wavelengths that are invisible to the eye. This predicted
the existence of what we now call radio waves.
In 1879, David E. Hughes, an
American music professor and scientific dabbler, discovered that a device called
an induction balance (something like a modern metal detector) caused noise in
his telephone, even when the phone was disconnected from the telephone network.
He assumed that Maxwell's invisible light, electromagnetic waves, traveled from
the induction balance to the telephone. He was living in England then, so he
took the opportunity to demonstrate his discovery to the Royal Society (the
ultimate authority of scientific knowledge at the time). However, at the time,
the scientific community did not accept Maxwell's predictions. The Royal Society
dismissed Hughes' discovery as mere induction, which was already well known at
the time.
In 1887, Heinrich Hertz produced radio waves in his
laboratory, measured their frequency and wavelength, and showed that they could
be refracted, reflected, and diffracted like other waves. In recognition of this
work, the unit of frequency is named the hertz (Hz). At one time,
electromagnetic waves were commonly called Hertzian waves.
Radio waves come from many
sources. Any object not at a temperature of absolute zero produces
electromagnetic radiation, some of which is in the frequency range called radio.
Stars, galaxies, nebulae, planets, rocks, rats, humans, etc., all produce
electromagnetic radiation. Radio waves are also produced by electric discharge,
such as lightning, automobile ignition systems, and the tiny spark produced in a
light switch.
Phase
Any wave consists of repeated cycles. For example, a voltage wave starts at zero volts, progresses to some maximum or peak value of positive voltage, returns to zero volts, progresses to some peak value of negative voltage then returns to zero volts again. That is one cycle, the wave being repeated cycles. The progress through the cycle is called the phase and is measured in degrees, a complete cycle being 360 degrees. Starting at zero volts, a voltage wave reaches the next peak 90 degrees later. It crosses zero volts at 180 degrees and reaches the opposite peak at 270 degrees. The cycle completes at 360 degrees.
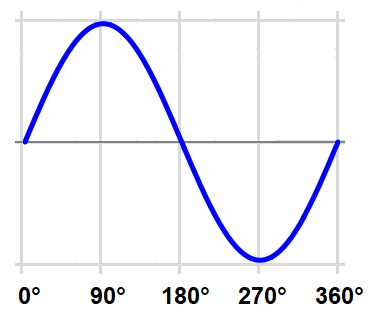 |
| One cycle of a sine wave with the phases labeled. |
Phase is often used to compare two waves of the same frequency occurring at different times. For example, one wave may reach zero volts at the time another wave reaches its peak voltage. These two waves are 90 degrees out of phase.
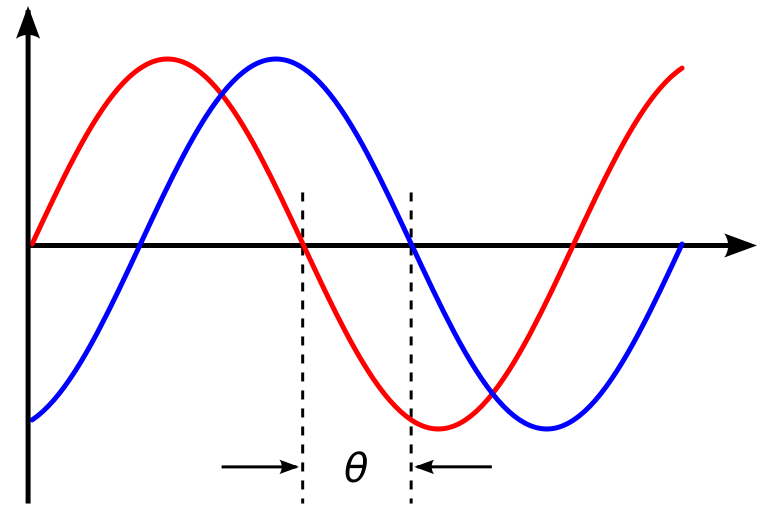 |
| Two sine waves approximately 80 degrees out of phase. The Greek letter theta labels the phase angle. |
If two such waves are mixed, the result is another wave with amplitude and phase between the two originals. If two waves that are 180 degrees out of phase are mixed, they mathematically subtract from each other, resulting in the difference between them. If such waves are equal in amplitude, they will cancel each other resulting in no wave after the mixing.
What are electromagnetic waves
The standard model of an electromagnetic wave is an oscillating electric field oriented at a right angle to an oscillating magnetic field. For example, an electromagnetic wave may be illustrated as an electric field oscillating up and down with a magnetic field oscillating from side to side (this is a vertically polarized wave, see below).
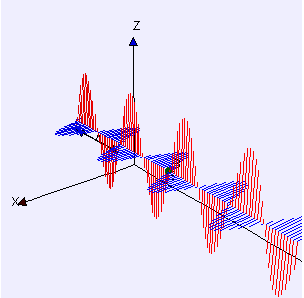 |
| Typical illustration of electromagnetic waves showing the magnitudes of the voltage and current and their orientation at right angles to each other |
This illustration is useful for depicting the
magnitude and orientation of the fields and the direction of travel but does not
give a useful picture of electromagnetic waves in free space. For example,
nothing moves up and down or from side to side, and the waves are not confined
to a finite-width "beam," as the illustration implies.
Imagine a still pool of water. The surface is flat and smooth as long as nothing
disturbs the water, such as wind or earthquakes. The water is in equilibrium.
Gravity pulls down on the water, hydrostatic pressure pushes up, and the surface
settles where the two forces are equal. Touch the water, and waves radiate away
from your finger; you disturbed the equilibrium. The effect of that
disturbance radiates away from where the disturbance happened.
The universe is made of a substance called spacetime. The nature of spacetime is still under investigation. However, if you disturb the equalibrum of spacetime you create electromagnetic waves. Like the surface waves on water just described, electromagnetic waves radiate away from the point of disturbance, except they radiate in three dimensions, like expanding bubbles of electromagnetism. Keep in mind that an electric field and a magnetic field are the same thing, just observed from different reletivistic frames of reference. Therefore, electromagnetic waves manifest as expanding spheres of electromagnetic fields where the electric and magnetic field are perpendicular to each other. These electromagnetic fields radiate like ripples in a pond at the speed of light.
|
|
|
An electromagnetic wave as an expanding spherical electric field. In this illustration, the voltage is higher as you go upward and lower as you go downward. Another expanding, spherical electric field is inside this one, with the positive pole at the bottom and the negative pole at the top. These keep expanding, one inside the other. |
When the next sphere reaches you, you will find that the polarity is reversed; the voltage is lower as you go up and higher as you go down. Each sphere has the opposite polarity to the one before it. If you are sufficiently far from the source of the electromagnetic waves, the fields will appear flat. It would be like wall after wall of electric fields passing by, each with polarity opposite to the previous one.
There is also an accompanying magnetic field mixed with the electric field. The poles of this magnetic field are perpendicular to the electric field and alternate with each passing wave. This magnetic field is not shown above to reduce clutter in the diagram. Furthermore, like all magnetic fields, this magnetic field is simply a product of the electric field with different perceived properties due to the effects of special relativity.
Polarization
The polarization of an electromagnetic wave is defined as the orientation of
the electric field. The electric field is oriented from pole to pole on the
spherically-shaped wave pulses. Therefore, if a wave is oriented such that the
electric poles are oriented vertically, the wave is said to be vertically
polarized. On the other hand, if the electric poles of the wave are oriented
horizontally, the wave is said to be horizontally polarized.
Electromagnetic waves were once thought to propagate through a substance called
"luminiferous ether." It was believed that this ether must exist because waves
must have something to propagate through. Experiments to detect this ether have
failed to produce evidence of its existence. However, spacetime is real, and
electromagnetic waves are affected by the spacetime they travel through. For
example, gamma rays produced by the Big Bang have expanded with the universe as
it expanded. These gamma rays have been stretched into radio waves approximately
2 mm in length over the billions of years since the Big Bang. This gamma-ray
"flash" from the Big Bang is now "visible" as a uniform "glow" of microwave
radiation that fills the sky (this is the cosmic microwave background or CMB).
Propagation
There are several different modes by which electromagnetic waves propagate.
Different frequencies are more efficient with some
modes than others.
Direct waves
The direct mode is line-of-sight. Direct waves go directly from the
transmitting antenna to the receiving antenna through free space.
Reflected waves
Reflected waves reflect off the ground or other objects. For example, very high
frequencies can reflect off buildings or aircraft.
Ground waves
Ground waves propagate
along the earth-air boundary, follow the earth's curvature, and are vertically
polarized. Sea water is more conductive than dry earth. Therefore, ground waves
propagate along the sea better than along dry earth. Ground waves are gradually
refracted into the earth, which limits their distance. Lower frequencies
propagate better than higher frequencies.
Sky waves
Sky waves are reflected off the
ionosphere. Different frequencies reflect off different layers of the
ionosphere. Some layers change their reflective properties between day and night
and are affected by solar activity. Some frequencies have their polarization rotated 90 degrees
or 180 degrees when reflected off the ionosphere. The distance a sky wave
reaches depends on which angle it hits the ionosphere and which layer off which
it reflects. There is often
an area between the transmitter and receiver that the sky wave skips over,
called the skip zone. Sky waves may bounce back and forth between the earth and
the ionosphere.
Tropospheric ducting
Tropospheric ducting traps electromagnetic waves between temperature inversion layers in the lower atmosphere. Weather conditions such as warm and cold fronts cause conditions conducent to tropospheric ducting.
Multipath reception
Reflected radio waves often cause a receiver to receive multiple signals from
the same transmitter that traveled different paths. If two paths are different
lengths, as they usually are, they will arrive out of phase. The result is that
the receiver receives a wave modified in amplitude, thus stronger or weaker than
the original.
This is a problem with wireless microphones in venues with
radio-reflecting surfaces, such as a performing stage. Since the transmitter
(microphone) is often moving, the receiver will receive multiple signals with
constantly changing phase relationships. The result is a signal that increases
and decreases in strength, causing the signal to be occasionally lost. This is
mitigated by using two antennas on the receiver. When the signals arrive out of
phase at one antenna, they usually arrive in phase at the other.
Multipath reception is also a problem with low-frequency long-distance
communication, such as commercial AM and shortwave broadcasting. A receiver may
receive the ground wave and the sky wave simultaneously. If these signals are
out of phase, the signal is attenuated. The reflective portion of the ionosphere
may move, changing the distance the sky wave travels and its phase compared to
the ground wave. The reflected wave may also have a random phase compared to the
original. A signal may also reflect off rain or even meteor trails. The receiver
may receive multiple signals from multiple changing paths. These factors often
cause a distant signal to fade in and out, called multipath fading.
Frequency and Wavelength
When waves move across
a body of water, you can see that the water's surface moves up and down as the
waves pass. The number of times this cycle repeats in one second is called the
frequency. With electromagnetic waves, the apparent electric and magnetic fields
increase and decrease in strength periodically, like surface waves. This is
accompanied by a periodic reversal of the electric and magnetic fields. The
frequency of an electromagnetic wave is defined as the number of times the
fields reverse polarity every second. Radio waves are electromagnetic waves with
frequencies from 20,000 cycles per second (20 kilohertz or 20kHz) to
300,000,000,000 cycles per second (300 gigahertz or 300GHz). The distance that a
wave travels during one cycle is the wavelength. With ocean waves, this is the
distance between crests (or troughs). As the frequency increases, it takes less
time to complete one cycle, so the waves travel a shorter distance in that time.
Therefore, higher frequency waves have a shorter wavelength. Electromagnetic
waves travel approximately 300,000,000 meters per second in a vacuum or air. At
this speed, radio waves at 30kHz have a wavelength of 10,000 meters. AM radio
waves, which have a frequency of about 1MHz, have a wavelength of about 300
meters. Radio waves with a frequency of 300GHz have a wavelength of 1 mm.
Electromagnetic waves with
frequencies below 20kHz may or may not be considered radio waves. They are
excluded above because these are in the audio frequency range in electronic
circuits. Circuits that work at these frequencies are considered audio frequency
(AF) circuits. Electromagnetic waves above 300GHz are in the infrared "light"
range. This is roughly the upper limit where electronic circuits can respond to
the oscillations or where antennas can create electromagnetic radiation.
The radio spectrum
Radio frequencies cover a wide range of frequencies. This range is broken up into several bands with different characteristics. The most commonly used designations are from the International Telecommunications Union (ITU). The band borders are based on wavelength in meters and consequently on frequencies in multiples of three (for example, a wavelength of one meter has a frequency of 300 MHz, and a wavelength of 10 meters has a frequency of 30 MHz). The following chart lists the various bands. In the chart below, the wavelength ranges are longer-to-shorter (e.g., 100 - 10m) because the wavelength gets shorter as you increase in frequency.
| Name | Start frequency |
wavelength at start frequency | Significant uses | Characteristics | |
| Tremendously low frequency |
TLF | 0 Hz |
infinite | ||
| Extremely low frequency |
ELF | 3 Hz |
100,000 km | Communication with submarines | Efficient ground wave propagation. Propagates below surface of water. |
| Super low frequency | SLF | 30Hz |
10,000 km | ||
| Ultra low frequency |
ULF | 300 Hz |
1,000 km | ||
| Very low frequency |
VLF | 3 kHz | 100 km | Communication with submarines, Time signals | |
| Low frequency |
LF | 30 kHz |
10 km | Longwave broadcasting, time signals, RFID, amateur radio | Efficient ground wave propagation |
| Medium frequency |
MF | 300 kHz | 1,000 m | AM Broadcasting (the AM broadcasting band is centered on 1 MHz which is displayed as 1,000 kHz on AM receivers in most regions), radio teletype, amateur radio | |
| High frequency |
HF | 3 Mhz | 100 m | Shortwave broadcasting, citizens band radio, amateur radio, RFID, Over-the-horizon radar, HF aircraft communication, marine, and mobile radio | Efficient sky wave propagation |
| Very high frequency |
VHF | 30 MHz | 10 m | FM broadcasting (the FM broadcasting band is centered on 100 MHz in most regions), television broadcasting, radar, aircraft communication and navigation, radioteletype, land mobile and maritime mobile communications, amateur radio, police, fire, and emergency medical services, weather radio, cordless phones. | Lower frequencies in this band have good sky wave propagation. Some also reflect off meteor trails. However, obstacles easily block higher frequencies, so those are line of sight (direct wave) only. Frequencies up to lower EHF can penetrate non-conductive walls. |
| Ultra high frequency |
UHF | 300 MHz | 1 m | Television broadcasting, microwave ovens, radars (L band), mobile phones, WiFi, Bluetooth, GPS, FRS, and GMRS radios, amateur radio, satellite radio, police, fire, and emergency medical services, remote control systems, ADS-B, cordless phones, communication satellites, weather satellites, satellite phones (L-band), satellite phones (S-band). | |
| Super high frequency |
SHF | 3 GHz | 10 cm | Microwave communications, Wifi, radar, communications satellites, amateur radio, weather satellites, satellite radio, cordless phones, satellite phones (S-band). | |
| Extremely high frequency |
EHF | 30 GHz | 1 cm | Satellite broadcasting, communication satellites, weather satellites, microwave radio relay, directed-energy weapons, WiFi 802.11ad. | |
| Tremendously high frequency |
THF | 300 GHz | 1 mm | Terahertz frequencies, AKA T-rays are being tested in medical imaging to replace X-rays, |
Wavelength and color
Electromagnetic waves at a frequency of about 600 THz (600,000,000,000,000 cycles per second) have a wavelength of about 500 nm (0.000,000,5 meter). This is near the middle of the visible spectrum and appears to humans as green light. The lower end of the visible spectrum is around 400 THz and a wavelength of 700 nm. The upper end of the visible spectrum is around 700 THz and a wavelength of 400 nm. The longer wavelengths are red, and the shorter wavelengths are violet. White light is a uniform mix of everything from 400 to 700 nm[3].
There is no color perception of radio waves, but when a broad range of radio waves are produced, it is sometimes said to be like white light. A color analogy can be useful when talking about the filtering of radio waves. Similarly, in acoustics, the term "white noise" describes a hissing sound with a uniform mix of frequencies over the entire audio spectrum.
What are Electromagnetic Waves
When EM Waves Have no Energy - Answers to Questions
—————————
| Vocademy |
